Generalized equation predicts hydrocarbon acentric factors
A new acentric factor correlation, based on Pitzer’s1 definition of the acentric factor and a correlation of Antoine’s equation,2 accurately predicts the acentric factor of hydrocarbons and other components.
This equation can be used in vapor pressure correlations and cubic equations of state to improve the prediction of physical properties of light and heavy hydrocarbons as well as other components.
The new general correlation predicts the acentric factor of hydrocarbons more accurately than other equations reported in the literature. The generalized equation presented here accurately estimates the acentric factor of hydrocarbon and other fluids using critical pressure, critical temperature, and normal boiling temperature, and it is independent of vapor pressure.
This article reports the application of the developed acentric factor equation in predicting vapor pressure and phase behavior of hydrocarbons.
Acentric factor correlations
The acentric factor is widely used to estimate the physical properties of hydrocarbons. Equations of state in general and, particularly, commonly used cubic equations of state are often used for heavy petroleum fractions because proper characterization of this fraction is very difficult. When an equation of state is applied to defined components or pseudopetroleum fractions, the value of acentric factor is needed to predict phase behavior.
In addition, one of the important areas of application of the acentric factor is the prediction of vapor pressure from the generalized vapor-pressure correlations. The Edalat (OGJ, Feb. 1, 1993, p. 39) and Lee-Kesler3 correlations, which predict the vapor pressure of hydrocarbons, are functions of the acentric factor.
Although many data in the literature include values of the acentric factor, ω, the value of ω for petroleum fractions is not readily available. Even for defined compounds, there are instances where experimentally determined acentric factor values are not available.
Vapor pressures, for example, may not have been measured at a reduced temperature, Tr, of 0.7 or the critical constants may simply be unavailable. Under these circumstances, the acentric factor cannot be calculated. One would therefore have to use estimation methods for the acentric factor or apply a general correlation of the acentric factor.
Although many estimation methods have been proposed, there are two practical ways to estimate ω from a single vapor-pressure point. One is to solve an acentric-dependent cubic equation of state and the other is to use a generalized vapor pressure equation. Both methods are lengthy and tedious procedures.
There are several equations that predict the acentric factor. Equation 1 (see attached equation box) shows Pitzer’s definition of the acentric factor, which represents the acentricity or nonsphericity of a molecule.1 He measured the deviation from simple molecules.
Equation 1 is in terms of the vapor pressure at a reduced temperature of 0.7 and the critical pressure. Prs = Ps/Pc, and is known as the reduced vapor pressure.
One must use the vapor pressure at a reduced temperature of 0.7 with Equation 1. Pitzer’s acentric factor is therefore inadequate for calculations performed in the critical region and for liquids at low temperatures.
Edmister (1986) proposed the following relation for the acentric factor based on two parameters of the Clausius-Clapeyron equation (Equation 2), where θ = Tb/Tc.4
Lee-Kesler developed a vapor pressure relation (Equation 3) for the acentric factor. Watanasiri, et al., also derived a very lengthy relation for acentric factor.5
We have developed an acentric factor correlation (Equation 4) that can be used in vapor-pressure correlations and cubic equations of state to improve the prediction of physical properties of light and heavy hydrocarbons. Our general correlation predicts the acentric factor more accurately than the other equations.
Our equation accurately estimates the acentric factor of hydrocarbon and other fluids using the properties of critical pressure, Pc, critical temperature, Tc, and normal boiling temperature, Tb. Contrary to Pitzer’s equation, our equation is independent of vapor pressure.
Generalized equation
Because the conventional characterization parameters of normal boiling point (NBP) and specific gravity are used in the refining industry, we used NBPs in our equation to estimate the acentric factor of petroleum fractions. Using the right correlation for calculating the critical temperature and pressure to determine Tr and Pc, one can then determine the acentric factor from the NBP.
Equation 4 shows the new generalized accurate correlation for acentric factor.
Equation comparability
We compared the acentric factors predicted from Equation 4 with those of Edmister (Equation 2) and Lee and Kesler (Equation 3). The results of calculated acentric factor from Equation 4 indicate that this equation can predict the acentric factor more accurately than the other acentric factor equations available in the literature.
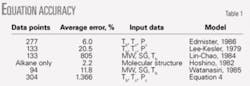
Table 1 shows the comparison between acentric factor of hydrocarbons using Edmister, Lee-Kesler, Lin-Chao,6 Hoshino,7 and Watanasiri correlations, and the proposed correlation of this article.
The new equation predicts the acentric factor accurately compared with other correlations in the literature. In addition, Table 1 shows the average percentage of errors of the new equation and other equations.
Acknowledgment
The authors thank the Faculty of Engineering of the University of Tehran.
References
- Pitzer, K.S., “The volumetric and thermodynamic properties of fluids, I-Theoretical basis and virial coefficients,” J. Am. Chem. Soc., Vol. 77 (1955), pp. 3427-32.
- Antoine, C., “Vapor pressure equation,” Combt. Rend., Vol. 107 (1883), p. 681.
- Kesler, L., and Sandler, “Third Parameter for Use in Generalized Thermodynamic Correlations,” Ind. Chem. Fund., Vol. 18 (1979), p. 49.
- Edmister, W.C., and Lee, B.I., Applied Hydrocarbon Thermodynamics, Gulf Publishing Co.: Houston, 1986, p. 173.
- Watanasiri, S., Owens, V.H., and Starling, K.E., “Correlations for estimating critical constants, acentric factor, and dipole moment for undefined coal-fluid fractions,” Ind. Eng. Chem. Process Des. Dev., Vol. 24 (1985), pp. 294-96.
- Lin, H.M., Chao, K.C., “Correlation of critical properties and acentric factor of hydrocarbons and derivatives,” AIChE J., Vol. 30 (1984), pp. 981-83.
- Hoshino, Nagahama, and Hirata, “Prediction of acentric factor of alkanes by the group contribution method,” J. Chem. Eng. Japan, Vol. 15 (1982), No. 2, pp. 153-55.
The authors
H.R. Hosseinbeigi works for the Oil & Energy Industries Development Co., Tehran.He holds a BSc and MSc, both in chemical engineering, from the University of Tehran.
Arabgol Fatemeh holds a BSc in chemical engineering from Isfahan University of Technology, Iran, and an MSc degree in chemical engineering from Tarbiat Modarres University, Iran.
Mohsen Edalat (MohEdalat @yahoo.com) is a professor of chemical engineering in the College of Engineering of the University of Tehran. He holds a BSc in chemical engineering from the University of Tehran and an MSc and PhD in chemical engineering from the University of Illinois.