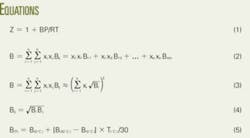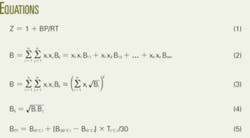Procedure calculates base gas compressibility factors
This article presents an easy and simple spreadsheet procedure for custody-transfer calculations of the base compressibility factor for any pair of pressure and temperature conditions.
The procedure uses the second virial coefficient data source from Reference 1. The calculated compressibility factors are similar with values obtained from the AGA 8 compressibility equation of state.2
Metering
In gas industry metering applications, the base (or standard) conditions for pressure and temperature vary, not only with the geographical region but among gas sales contracts.
In the US, the common base or standard reference conditions are 60º F. and 14.73 psia. In Europe, the normal reference conditions are 0º C. and 101.325 kPa, while the standard conditions are 15° C. and 101.325 kPa. Argentina also uses 15º C. and 101.325 kPa, while Mexico uses 20º C. and 1 kg/sq cm absolute.
Calculations of real gas heating value, density, base density, base volume, and Wobbe index require compressibility factors at base conditions. Tables list these properties but only as ideal gas values.
For custody-transfer, the procedure has to adjust these values by including a calculated compressibility factor at the appropriate base conditions.
References provide data tables and methods for obtaining the compressibility factor at base conditions, such as GPA 2145, although these data generally are only true for one of most used base conditions, which are 60º F. and 14.73 or 14.696 psia.
Anyone using other base conditions has to find another method besides that provided by the tables.
One solution is to use AGA 8 program (last version), but this option requires complex algorithms and time-consuming computer programming or third-party software.
This article presents a spreadsheet procedure to calculate, at custody-transfer-level requirements, a compressibility factor at base conditions for any pair of pressure and temperature conditions.
Compressibility factor
At conditions near ambient, pressure less than 16 psia, the truncated virial equation of state (Equation 1 in the equation box) satisfactorily represents the volumetric behavior of natural gas.
In Equation 1, the nomenclature is:
- Z = Compressibility factor at base conditions.
- B = Second virial coefficient.
- R = Gas constant
- P = Absolute pressure at base condition.
- T = Absolute pressure at temperature condition.
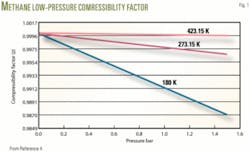
For natural gas, the compressibility factor value at base conditions is nearly 1, such as 0.99, so that B has to be negative (Fig. 1).
Equation 2 shows the second virial coefficient for a mixture. In the equation, Bij = Bji is the second interaction (cross) virial coefficient for component i and j, and Bii is second virial coefficient for pure component i. The second virial coefficients are functions of temperature.
Equation 3 provides an alternative expression for B that is more convenient for hand calculations, is satisfactory for custody-transfer applications, and is more useful and clearer. In the equation the square root of Bi is the summation factor, a common term found in the literature.1 3 4
Values for the summation factor at 60º F. appear frequently in tables, but there are no tables that list summation factors for other base temperatures. That is the problem and the goal of this article is to present a procedure to obtain the compressibility factor at any temperature.
This procedure does not use the summation-factor method but the second virial coefficient that is more useful and clearer.
Equation 3 assumes that Equation 4 is correct. Two examples explain the use of this equation.
Methane (B1) and ethane (B2) have B factors at 0° C. of -53.28 and -219.38, respectively. A methane and ethane mixture (B12) would than have a B factor that is the square-root of the product of B1 times B2 (Equation 4) or -108.11. Experimental datum is -111.86, which is close enough for practical purposes.5
Propane (B3) has a B factor at 0° C. of -470. A methane and propane mixture (B13) than would have a B factor (Equation 4) of -158. Experimental datum is -156.5
Equation 4 is the key for obtaining the simplified expression in Equation 3 and reducing the number of variables in the calculation.
Table 1 lists the second virial coefficients of pure hydrocarbon and inert gases in custody range pressures and temperatures.
DIN 1871 includes data only for two temperatures (0º C. and 30º C.), but says that linear interpolation with Equation 5 can provide the values for other temperatures.
B values in Table 1 for other temperatures were calculated in this manner. This procedure can be used for any base temperature.
Table 2 shows calculations for two gas mixtures at 0° C. and 60° F. at a base pressure less than 16 psia
References
- DIN 1871, Gaseous fuels and other gases-Density and other Volumetric quantities, 1980.
- Report No. 8, AGA, Compressibility Factors of Natural Gas and Other Related Hydrocarbon Gases, 1992.
- GPA 2172 (API MPMS 14.5), Calculation of Gross Heating Value, Relative Density and Compressibility Factor for Natural Gas mixtures from Compositional Analisys, 1996.
- NCh 2380 (ISO 6976), Gas Natural-Cálculo del Poder Calorífico, Densidad, Densidad Relativa y Numero de Wobbe a partir de la composición, 1997.
- Dymond, J.H., and Smith, E.B., The Virial Coefficients of Pure Gases & Mixtures, Oxford University Press, New York, 1980.
The author
Nelson Menendez ([email protected]) is an operations engineer and project leader for natural gas production at Empresa Nacional del Petróleo (ENAP), Magallanes, Chile. He also is the custody transfer project leader for ENAP innovative solutions program. Menendez holds a chemical (petrochemical) engineering degree from a local university and a 1-year diploma in professional and business development and project management.